Question

A. f(x) = 2^x +3
B. f(x) = 3^x-5
C. f(x) = (1/2)^x +5
D. f(x) = (1/2)^x

Answer 1

Answer: D

Explanation:

The given graph represents "exponential decay", meaning as the graph graph moves left-to-right (or the values of x go from negative to positive), the values get smaller (or the value of y decreases).

Exponential functions are modeled by the following:

`y = a^(x) + b`

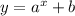
If the value of a is greater than 1, then the function exponentially grows (you can see this by setting a=2 and putting in larger, and larger values for x to see the resulting y value grow), and if the value of a is less than 1, then the function exponentially decays.

Since the graph represents exponential decay, that means a must be less than 1, so we can rule out answers A and B, which leaves us with C and D.

When x=0, according to the graph, the value of y is 1, so if we plug in x=0 for answers C and D, we know that any number raised to the power of 0 is 1, so that leaves us with 6 and 1, respectively, for C and D, meaning the answer is D.