Question

The two statements below are different ways to say that the sides of these similar triangles are proportional.

Complete the equations that go with the statements.


PLSS I NEED THISS

Answer 1

• e, c
• c, d

Explanation:

You want the different ways sides of similar triangles can be shown as proportional.

Corresponding sides

From shorter to longer, the corresponding sides in the similar triangles are ...

Smaller ... Larger

b . . . . . . . e

a . . . . . . . d

c . . . . . . . f

Pairs

The proportions are written by choosing two letters and their corresponding letters. The two chosen can be from different columns (part 1) or from the same column (part 2). The corresponding letters are written in the same place in the proportion fraction

`(a)/(d)=\frac{b}{\boxed{e}}=\frac{\boxed{c}}{f}`

`(a)/(b)=(d)/(e)\qquad\frac{b}{\boxed{c}}=(e)/(f)\qquad(c)/(a)=\frac{f}{\boxed{d}}`

__

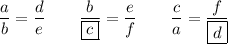
Additional comment

Any proportion can be written "sideways" or "upside down." Corresponding parts have the same relationship in any case.

`(a)/(b)=(d)/(e)\ \Leftrightarrow\ (a)/(d)=(b)/(e)\ \Leftrightarrow\ (b)/(a)=(e)/(d)\ \Leftrightarrow\ (d)/(a)=(e)/(b)`