Answer:
B. ∠b ≅ ∠g
Explanation:
Supplementary angles
Two angles whose measures sum to 180°.
Linear Pair
Two adjacent angles that sum to 180° (two angles which when combined together form a straight line).
Same-side Interior Angles Theorem
When two parallel lines are intersected by a transversal, the angles that are interior to the parallel lines and on the same side of the transversal line are supplementary (sum to 180°).
Vertical Angles Theorem
When two straight lines intersect, the opposite vertical angles are congruent.
------------------------------------------------------------------------------------------
Statement A
∠f and ∠h are vertical angles. Therefore, according to the Vertical Angle Theorem, ∠f ≅ ∠h.
Statement C
As ∠d and ∠h are the interior angles on the same side of the transversal
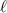 , according to the Same-side Interior Angles Theorem, ∠d and ∠h are supplementary.
, according to the Same-side Interior Angles Theorem, ∠d and ∠h are supplementary.
Statement D
∠a and ∠b are a linear pair, therefore ∠a and ∠b are supplementary.
Therefore, Statement B cannot be supported by the evidence shown.