Answer:
Option B.
Explanation:
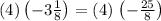
Next, remove the brackets.
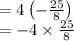
Then, convert 4 into a fraction.
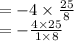
Multiply the bottom numbers then factor.
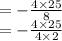
Now, in order to continue, you have to cancel the common factor, 4.
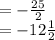
Why option B is the same as the original equation is because it's the same equation with more steps.
Option B:
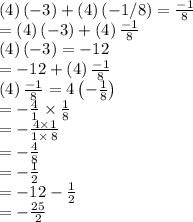
And 25 halves is 12 and a half, so this is why option B is identical.
Hope this helps!