Find the remainder when x^100 - x^99 + x^98 − · · · − x + 1 is divided by x + 1.

Question

asked Mar 21, 2022 184k views

2 Answers

Daan Wilmer · Answer 1 · 2022-03-25T18:01:48+0000

Answer:

The remainder is 101.

Explanation:

We are dividing:

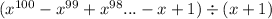
(x^(100)-x^(99)+x^(98)...-x+1)/(x+1)

By the Polynomial Remainder Theorem, if we are dividing a polynomial P(x) by a binomial in the form (x - a), the our remainder will be P(a).

The divisor is (x + 1). Therefore, our a = -1.

Then the remainder will be:

P(-1)

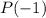
We can rewrite our polynomial as:

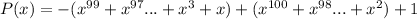
P(x)=-(x^(99)+x^(97)...+x^3+x)+(x^(100)+x^(98)...+x^2)+1

Each of the parentheses contain fifty terms. -1 to any odd power is -1, and -1 to any even power is 1. Therefore:

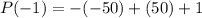
P(-1)=-(-50)+(50)+1

Evaluate:

P(-1)=101

The remainder is 101.