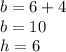Answer:
Base = 10 cm
Height = 6 cm
Explanation:
Here is the formula for the area of a triangle.
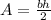
We are given
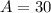
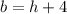
Replace all occurrences of
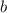 with
with
 in each equation.
in each equation.
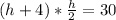
Apply the distributive property.
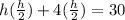
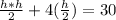
Use the power rule to combine exponents.
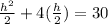
Factor 2 out of 4 .
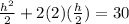
Cancel the common factor.
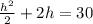
Solve for h.
Multiply each term in
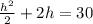 by 2 to eliminate the fractions.
by 2 to eliminate the fractions.
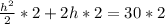
Simplify each term.
Cancel the common factor of 2.
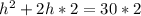
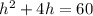
Subtract 60 from both sides of the equation.
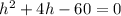
Factor using the AC method.
Consider the form
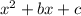 . Find a pair of integers whose product is
. Find a pair of integers whose product is
 and whose sum is
and whose sum is
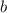 . In this case, whose product is − 60 and whose sum is 4 .
. In this case, whose product is − 60 and whose sum is 4 .
−6, 10
Write the factored form using these integers.
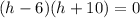
Set
 equal to 0 and solve for h.
equal to 0 and solve for h.
Then add 6 to both sides of the equation.
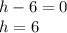
Set
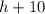 equal to 0 and solve for h.
equal to 0 and solve for h.
Then subtract 10 from both sides of the equation.
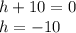
The final solution is all the values that make
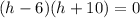 true.
true.
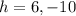
Given
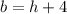
Replace all occurrences of h with 6 in each equation.