Question

Find a quadratic polynomial with integer coefficients which has x = 2/9, ± SQRT23/9 as its real zeros.

Answer 1

The quadratic polynomial with integer coefficients is
`y = 81\cdot x^(2)-36\cdot x -19`.

Explanation:

Statement is incorrectly written. Correct form is described below:

Find a quadratic polynomial with integer coefficients which has the following real zeros:
`x = (2)/(9)\pm (√(23))/(9)`.

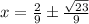Let be
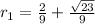`r_(1) = (2)/(9)+(√(23))/(9)` and
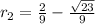`r_(2) = (2)/(9)-(√(23))/(9)` roots of the quadratic function. By Algebra we know that:

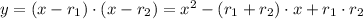`y = (x-r_(1))\cdot (x-r_(2)) = x^(2)-(r_(1)+r_(2))\cdot x +r_(1)\cdot r_(2)` (1)

Then, the quadratic polynomial is:

`y = x^(2)-(4)/(9)\cdot x -(19)/(81)`

`y = 81\cdot x^(2)-36\cdot x -19`

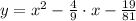
The quadratic polynomial with integer coefficients is
`y = 81\cdot x^(2)-36\cdot x -19`.