Question

hope has been tracking the progress on her family as they travel across the country she know they are driving 78 miles per hour during their vacation and she created a function d(t) =78t to model the process they are making. how many hours will it take for them to be a total distance of 390 miles from their starting point hurry for 100 points

Answer 1

5 hours

Explanation:

The function d(t) = 78t represents the distance traveled (d) as a function of time (t), where the speed is constant at 78 miles per hour.

To find out how many hours it will take to travel a total distance of 390 miles, we can substitute d(t) = 390 into the function:

`78t = 390`

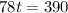
Solve for t by dividing both sides of the equation by 78:

`(78t)/(78)=(390)/(78)`

`t=5\; \sf hours`

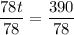
Therefore, it will take 5 hours for Hope's family to be a total distance of 390 miles from their starting point, assuming they maintain a constant speed of 78 miles per hour.

Answer 2

5 hours

Explanation:

To find the time (t) it takes for them to travel a total distance of 390 miles, we can use the given function d(t) = 78t.

Set d(t) equal to 390 and solve for t:

`\sf d(t) = 78t`

`\sf 390 = 78t`

Now, divide both sides by 78:

`\sf (390)/(78) = (78t)/(78)`

Simplify the fraction:

`\sf 5 = t`

`\sf t = 5`

So, it will take them 5 hours to travel a total distance of 390 miles from their starting point.