Answer:
1) The monthly payment is approximately $292.96
2) The total interest is approximately $2,061.84
Explanation:
1) The financing method Nathan Reynolds applied to buy the car is given as follows;
The purchase price of the car = $15,000
The down payment made for the car = $3,000
The
The interest on the loan he took for the balance of the purchase price, R = 8%
The number of years for which he took the loan, n = 4 years = 48 months
We have;
The balance of the purchase price = (The purchase price of the car) - (The down payment made for the car)
∴ The balance of the purchase price = $15,000 - $3,000 = $12,000
The balance of the purchase price = $12,000 = The loan Nathan secured
The monthly payment on a loan is given by the following formula
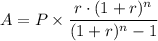
Where;
A = The payment amount made monthly
P = The principal or loan amount = $12,000
r = The interest rate divided by 12 = R/12 = 8/12 = 2/3
n = The number of months the monthly payment will be made = 48 months
By plugging in the variable values, we get;

Therefore, the payment amount made monthly, A ≈ $292.96
2) The total payment made in the 48 months = A × 48 = 292.955068099 × 48 ≈ 14,061.84
The total payment made in the 48 months ≈ $14,061.84
The total interest = (The total payment made in the 48 months) - (The loan amount)
∴ The total interest = $14,061.84 - $12,000 ≈ $2,061.84