Question

In AOPQ, o = 700 cm, p = 840 cm and q=620 cm. Find the measure of _P to the
nearest degree

Answer 1

79

Explanation:

Answer 2

Given:

In triangle OPQ, o = 700 cm, p = 840 cm and q=620 cm.

To find:

The measure of angle P.

Solution:

According to the Law of Cosines:

`\cos A=(b^2+c^2-a^2)/(2bc)`

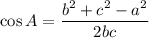
Using Law of Cosines in triangle OPQ, we get

`\cos P=(o^2+q^2-p^2)/(2oq)`

`\cos P=((700)^2+(620)^2-(840)^2)/(2(700)(620))`

`\cos P=(490000+384400-705600)/(868000)`

`\cos P=(168800)/(868000)`

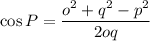
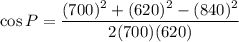
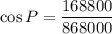
On further simplification, we get

`\cos P=0.19447`

`P=\cos^(-1)(0.19447)`

`P=78.786236`

`P\approx 79`

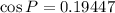
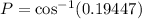
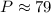
Therefore, the measure of angle P is 79 degrees.