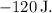The work done by gravity moving a 2 kg object from O to B, given a gravitational field of 10 N/kg, is -120 J. The negative sign indicates work against gravity.
The work done by gravity as an object moves in a gravitational field is given by the formula:
![\[ W = -mgh \]](https://img.qammunity.org/2024/formulas/physics/high-school/u7kfnx3c5n7egsz8fyqv0mm14mcmyypdiy.png)
where:
-
 is the work done,
is the work done,
- m is the mass of the object,
- g is the gravitational field strength,
- h is the vertical displacement.
In this case, the gravitational field is given as
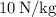 in the -y direction, and the object is moved along a path in the xy-plane. The displacement vector
in the -y direction, and the object is moved along a path in the xy-plane. The displacement vector
 is parallel to the gravitational field, so the work done is given by:
is parallel to the gravitational field, so the work done is given by:
![\[ W = -mgh \]](https://img.qammunity.org/2024/formulas/physics/high-school/u7kfnx3c5n7egsz8fyqv0mm14mcmyypdiy.png)
Since the gravitational field is
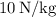 , and the mass
, and the mass
 we can calculate
we can calculate
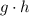 using the vertical displacement
using the vertical displacement
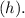
The vertical displacement
 is the difference in y-coordinates between the initial and final positions of the object.
is the difference in y-coordinates between the initial and final positions of the object.
1. From O to A:
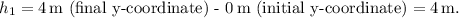
2. From A to B:
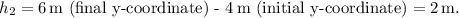
Now, calculate the total vertical displacement
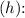
![\[ h = h_1 + h_2 = 4 \, \text{m} + 2 \, \text{m} = 6 \, \text{m} \]](https://img.qammunity.org/2024/formulas/physics/high-school/ba9ubl43q80prihp5te35rslfy7qklsf5i.png)
Now, substitute the values into the formula:
![\[ W = -mgh = -(2 \, \text{kg})(10 \, \text{N/kg})(6 \, \text{m}) \]](https://img.qammunity.org/2024/formulas/physics/high-school/hgihom83uah4ju9muil0hw1ga563u07r8t.png)
![\[ W = -120 \, \text{J} \]](https://img.qammunity.org/2024/formulas/physics/high-school/pyw4osdp7yoyrncept0z9fcr8hen29w82c.png)
The negative sign indicates that the work is done against the gravitational field, which makes sense because the object is moving in the positive y-direction. Therefore, the correct answer is