Question

The speed of a particle moving along the x axis of the (x, y) plane is V - (14-8t+t²) m/s where t is in seconds. Calculate the average acceleration from t = 1 sto t = 4 s.

Answer 1

The average acceleration of the particle from t = 1 s to t = 4 s is calculated by using the change in velocity over the change in time, which yields an average acceleration of -3 m/s².

The question asks to compute the average acceleration of a particle based on its velocity function which changes with time along the x-axis. The function for velocity is given as V = (14 - 8t + t²) m/s, where t is the time in seconds. To find the average acceleration, we need to look at the change in velocity over a certain time period, which is from t = 1 s to t = 4 s.

First, calculate the velocity at t = 1 s and t = 4 s:

Velocity at
`t = 4 s: V(4) = 14 - 8(4) + (4)² = 14 - 32 + 16 = -2 m/s`

`\Delta V = V(4) - V(1) = -2 m/s - 7 m/s = -9 m/s`

`\Delta t = 4 s - 1 s = 3 s`

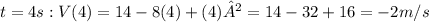
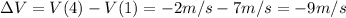
Now, compute the average acceleration (aavg) using the formula:

`a_(avg) = \Delta V / \Delta t = (-9 m\s)/(3s) = -3 m/s^2`

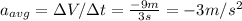
Therefore, the average acceleration of the particle from t = 1 s to t = 4 s is -3 m/s², meaning it is accelerating in the negative x-direction.