Question

Which equation represents a line which is perpendicular to the line y= -1/2x - 7?

a. 2x + y = 2 c. x - 2y = 6
b.y - 2x = 4 d. x + 2y = -8

(GIVING 100 POINTS!)

Answer 1

b) y-2x=4

Explanation:

To find the equation of a line perpendicular to y = -1/2x - 7, we need to consider the slope:

Original line slope: -1/2 (remember, negative sign for perpendicularity)
Perpendicular line slope: The negative reciprocal of -1/2 is 2.
Therefore, the perpendicular line will have a slope of 2.

b) y - 2x = 4

Rewrite in slope-intercept form: y = 2x + 4
Slope is 2, matches! This could be the answer.

Answer 2

b) y - 2x = 4

Explanation:

To find the equation of a line perpendicular to
`\sf y = -(1)/(2)x - 7`, we need to consider the slope.

The given line has a slope of
`\sf -(1)/(2)`, so a line perpendicular to it will have a slope that is the negative reciprocal of
`\sf -(1)/(2)`, which is
`\sf 2`.

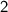Now, let's examine the answer choices:

a.
`\sf 2x + y = 2` - Not perpendicular (slope is
`\sf -2`).

b.
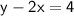`\sf y - 2x = 4` - This is perpendicular because the slope is
`\sf 2`.

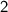c.
`\sf x - 2y = 6` - Not perpendicular (slope is
`\sf (1)/(2)`).

d.
`\sf x + 2y = -8` - Not perpendicular (slope is
`\sf -(1)/(2)`).

So, the equation representing a line perpendicular to
`\sf y = -(1)/(2)x - 7` is:

`\sf y - 2x = 4`

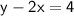
Therefore, the correct answer is (b).