Question

The diagram of the track on the field is show. below. It consists of two parallel lines and a semicircle at each end. The track is 10 meters wide. What is the area of the track?

Answer 1

The area of the track is approximately 3604.77 m²

What is the area of the track?

The area of the track can be calculated by finding the area of the two semicircles at the ends and the rectangular part in the middle, and then adding them together. The formula for the area of a track with two semicircles is:

A = πr² + lr

Where:

r = radius of the semicircle

l = width of the track

π is a constant approximately equal to 3.14159

Given that the track is 10 meters wide and the radius of each semicircle is 32 meters, we can calculate the area as follows:

Area of the two internal semicircles:

`A_(semicircle) = \pi r^2`

`A_(semicircle) = \pi * 32^2`

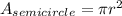
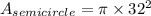
`A_(semicircle)` = 3217 m²

Area of the rectangular part:

`A_(rectangle,internal)` = 100 * 64 = 6400 m²

Total internal area = 6400 m² + 3217 m²

Total internal area = 9617 m²

Area of the two external semicircles:

`A_(semicircle) = \pi r^2`

`A_(semicircle) = \pi * 42^2`

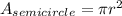
`A_(semicircle)` = 5541.77 m²

Area of the rectangular part:

`A_(rectangle,external)` = 120 * 64 = 7680 m²

Total external area = 5541.77 m² + 7680 m²

Total external area = 13221.77 m²

Total area of the track:

13221.77 m² - 9617 m² = 3604.77 m²

So, the area of the track is approximately 3604.77 m²