Question

The value of a certain investment over time is given in the table below. Answer the questions below to explain what kind of function would better model the data, linear or exponential.

Answer 1

An exponential function would best fit the data because as x increases, the y values change multiplicatively. The common ratio of this function is approximately 0.8.

In Mathematics and Geometry, an exponential function can be modeled by using this equation:

`f(x)=a(b)^x`

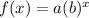
Where:

• a represents the initial value or y-intercept.
• x represents x-variable.
• b represents the rate of change or common ratio.

Generally speaking, multiplicative rate of change is peculiar to an exponential function and it refers to a numerical value that each of its consecutive y-value are multiplied by.

An exponential function has a common ratio and this can be calculated as follows;

Common ratio =
`(y_2)/(y_1)`

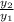
Common ratio = 23,048.91/27,156.71 ≈ 18,932.31/23,048.91 ≈ 14820.01/18,932.31

Common ratio ≈ 0.8.