According to the information provided, triangle ABC cannot be constructed (option E).
Let's use the Law of Sines to find the measure of angle B in triangle ABC.
The Law of Sines states:
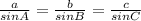
Given:
A=68
a=14,
c=17
We have a=14 and c=17. We need to find angle
B, and then we can find angle
C using the fact that the sum of angles in a triangle is 180
Let's find angle B using the Law of Sines:
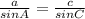
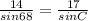
First, find sin68∘ :
sin68 ≈0.927
Now, solve for sinC:
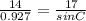
sinC≈ 17×0.927/ 14
sinC≈1.123
However, sinC cannot be greater than 1, which means the given information doesn't create a valid triangle. Therefore, according to the information provided, triangle ABC cannot be constructed (option E).