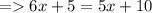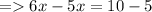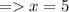Question

`4 * 8 ^(2x + 1?) = 32 ^(x + 2)`
​

Answer 1

x = 5

Explanation:

__________________________________________________________

FACTS TO KNOW BEFORE SOLVING :-

• 
  `a^x * a^y = a^(x+y)`

• In an equation , if the bases are same in both L.H.S. & R.H.S. then , the power of the bases on both the sides of equation should be equal. For e.g. :
  `a^x = a^y` ⇒
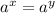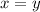  `x = y` [∵ Bases are equal on both the sides]

__________________________________________________________

`4 * 8^(2x+1) = 32^(x+2)`

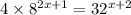
Lets express it in terms of 2.

`=> 2^2 * 2^(3 (2x+1)) = 2^(5(x+2))`

`=> 2^2 * 2^(6x+3) = 2^(5x+10)`

`=> 2^(2 + 6x+3) = 2^(5x+10)`

`=> 2^(6x+5) = 2^(5x+10)`

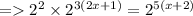
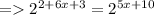
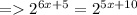
Here the bases on both the sides are equal. Hence ,

`=> 6x + 5 = 5x + 10`

`=> 6x - 5x = 10 - 5`

`=> x = 5`