The correct answer is 81.44.
To calculate the interest earned on the Certificate of Deposit (CD), we can use the compound interest formula:
![\[A = P \left(1 + (r)/(n)\right)^(nt)\]](https://img.qammunity.org/2024/formulas/business/high-school/qbt64n0zcusl0cr29qewk9ldkubuogsf2v.png)
Where:
-
 is the future value of the investment/loan, including interest.
is the future value of the investment/loan, including interest.
-
 is the principal amount (initial deposit).
is the principal amount (initial deposit).
-
 is the annual interest rate (as a decimal).
is the annual interest rate (as a decimal).
-
 is the number of times that interest is compounded per unit \(t\).
is the number of times that interest is compounded per unit \(t\).
-
 is the time the money is invested or borrowed for in years.
is the time the money is invested or borrowed for in years.
In this case:
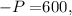

 (compounded monthly),
(compounded monthly),
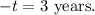
![\[A = 600 \left(1 + (0.0425)/(12)\right)^(12 * 3)\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/gk1d2elju88z5hivp1isrbbtf7tdz76ufu.png)
Calculating this will give us the future value of the investment after 3 years. To find the interest earned, subtract the initial deposit
 from the future value
from the future value
 :
:
![\[Interest = A - P\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/9olwv2rf9s0khcyuijvektpasbmwbea450.png)
Performing the calculations, the interest earned is approximately $81.44. Therefore, the correct answer is 81.44.