The measure of ∠A to the nearest 10th of a degree is 59.34 degree
How can the angle be calculated?
Trigonometry is the area of mathematics that deals with particular angle functions and how to use them in computations. In trigonometry, an angle can have six common functions. The terms sine (sin), cosine (cos), tangent (tan), cotangent (cot), secant (sec), and cosecant (csc) are their names and abbreviations.
Using the cosine rule
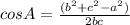
Where
a = 7.4 cm
b = 4.6 cm
c=8.6 cm
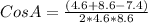
=
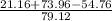
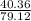
Cos A =0.510
Angle A =
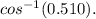
=59.336170257614 °
=59.34degree