Question

the speed of all electromagnetic waves in empty space is 3.00 x 10^8 m/s. calculate the wavelength of electromagnetic waves emitted at radio waves at 88.0 MHz

Answer 1

Approximately
`3.41\; {\rm m}`.

Step-by-step explanation:

The wavelength
`\lambda` of a wave can be found by dividing the wave speed
`v` by the frequency
`f` of the wave:

`\displaystyle \lambda = (v)/(f)`.

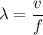In this question, it is given that:

• 
  `v = 3.00 * 10^(8)\; {\rm m\cdot s^(-1)}`.
• 
  `f = 88.0\; {\rm MHz}`.

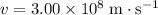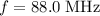Note the unit conversion:

• 
  `1\; {\rm Hz} = 1\; {\rm s^(-1)}`.
• 
  `1\; {\rm MHz} = 10^(6)\; {\rm Hz} = 10^(6)\; {\rm s^(-1)}`.

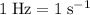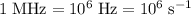Hence:

`f = 88.0* 10^(6)\; {\rm s^(-1)}`.

Using the equation
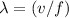`\lambda = (v / f)`, the wavelength of this wave (in vacuum) would be:

`\begin{aligned} \lambda &= (v)/(f) \\ &= \frac{3.00 * 10^(8)\; {\rm m\cdot s^(-1)}}{88.0* 10^(6)\; {\rm s^(-1)}} \\ &\approx 3.41\; {\rm m}\end{aligned}`.

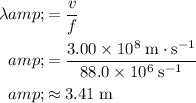In other words, the wavelength of this wave would be approximately
`3.41\; {\rm m}` in vacuum.