Answer:
y = 1x + -5
Explanation:
slope-intercept form is y = mx + b, where m = slope and b = y-intercept.
the line touches the y-axis at -5, therefore the y-intercept, or b, is -5.
y = mx + b ⇒ y = mx - 5
now i'll find the slope by picking two points from the line and finding the change in y over the change in x, aka
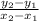 . i'll use the points (8, 3) and (5, 0) from the line in order to calculate the slope. (8, 3) can be my
. i'll use the points (8, 3) and (5, 0) from the line in order to calculate the slope. (8, 3) can be my
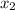 and
and
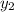 and (5, 0) can be my
and (5, 0) can be my
 and
and
 .
.
first, i'm going to plug in my
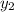 and
and
 values, which are the y-values from each of the coordinate pairs. since i picked (8, 3) for
values, which are the y-values from each of the coordinate pairs. since i picked (8, 3) for
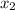 and
and
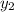 and (5, 0) for
and (5, 0) for
 and
and
 , i will be plugging in 3 for
, i will be plugging in 3 for
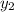 and 0 for
and 0 for
 .
.
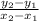 ⇒
⇒
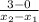
then i'll plug in my
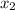 and
and
 values, which will be 8 and 5.
values, which will be 8 and 5.
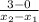 ⇒
⇒
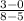
now simplify by subtracting 0 from 3 and 5 from 8.
the slope is
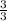 , which simplifies to 1 because
, which simplifies to 1 because
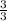 or 3 ÷ 3 = 1.
or 3 ÷ 3 = 1.
the slope-intercept form equation for this line is y = 1x + -5 (or just y = x - 5, but since it's a fill-in-the-blank problem, the 1 will show in front of the y and -5 will come after the + sign.)
i hope this helps! have a lovely day <3