Question

Some biologists believe the evolution of handedness is linked to complex behaviors such as tool-use. Under this theory, handedness would be genetically passed on from parents to children. That is, left-handed parents would be more likely to have lefthanded children than right-handed parents. An alternate theory asserts that handedness should be random, with left- and right-handedness equally likely. In a recent study using a simple random sample of n  76 right-handed parents, 50 of the children born were right-handed. ( pˆ =0.658 .) Suppose handedness is a random occurrence with either hand equally likely to be dominant, implying that the probability of a right-handed offspring is p =0.50.

Required:
a. Show that it is reasonable to approximate the sampling distribution of p̂ using a normal distribution.
b. Assuming left- and right-handed children are equally likely from right-handed parents, what is the probability of observing a sample proportion of at least p̂ =0.658 ?

Answer 1

a) Since both np >= 5 and n(1-p) >= 5, it is reasonable to approximate the sampling distribution of p^‚ using a normal distribution.

b) 0.5 = 50% probability of observing a sample proportion of at 0.658.

Explanation:

a. Show that it is reasonable to approximate the sampling distribution of p̂ using a normal distribution.

We need that:

np >= 5

n(1-p) >= 5

Sample of 76, 50 of the children were born were right-handed.

So
`n = 76, p = (50)/(76) = 0.658`

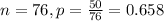
np = 76*0.658 >= 5

n(1-p) = 76*0.342 >= 5

Since both np >= 5 and n(1-p) >= 5, it is reasonable to approximate the sampling distribution of p^‚ using a normal distribution.

b. Assuming left- and right-handed children are equally likely from right-handed parents, what is the probability of observing a sample proportion of at least p̂ =0.658 ?

This is the mean, so 50% below and 50% above.

0.5 = 50% probability of observing a sample proportion of at 0.658.