Answer:
- initial speed is final speed: 0
- maximum speed is about 5.273 m/s
- distance A to B is 50 meters
Explanation:
You have a particle whose acceleration is a(t) = 3t²/160 -t³/800 and whose velocity at t=20 is 0. You want to show the initial speed is 0, find the maximum speed, and the distance traveled in 20 seconds.
(i) Initial speed
The speed is the sum of the initial speed and that due to acceleration. The speed due to acceleration is the integral of acceleration:
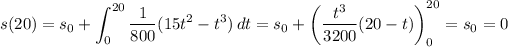
The change in speed over 20 seconds is zero, so the final speed is the initial speed. The final speed is zero, so the initial speed is 0.
(ii) Maximum speed
The maximum speed is where the acceleration is zero.
a(t) = 0 = (t²/800)(15 -t)
The acceleration is zero at t=0 and at t=15.
From the above integral, the maximum speed is ...
s(15) = 15³(20 -15)/3200 = 5.2734375 m/s ≈ 5.27 m/s
(iii) Distance
The distance traveled is the integral of the speed.
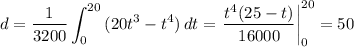
The particle traveled 50 meters from A to B.