Final answer:
To find the point on the operating characteristic curve for a lot proportion of defectives of 0.06 with an acceptance number of 2, we use the hypergeometric distribution and calculate the sum of probabilities of 0, 1, and 2 defectives in a sample of 50 using the binomial distribution formula.
Step-by-step explanation:
The student is asking about calculating a point on the operating characteristic curve (OC curve) for a given lot proportion of defectives, which is a concept in quality control and statistical sampling. When the lot size, N, is infinite, and a specified acceptance number is given, the point on the OC curve corresponds to the probability that a randomly selected sample of given size will have defectives not exceeding the acceptance number. The acceptance number in this case is 2, the sample size is 50, and the lot proportion of defectives, p, is 0.06.
To calculate the required probability, we use the hypergeometric distribution since the lot size is infinite, and hence the binomial distribution can be used. The formula to calculate the probability of exactly x defectives in a sample of size 50 when the lot proportion of defectives is 0.06 is:
P(X = x) = [C(n, x) * C(N-n, r-x)] / C(N, r)
Here, X is the random variable representing the number of defectives, n is the sample size (50), N is the lot size (infinite, so we use binomial approximation), r is the total number of defectives in the lot, and x is the number of defectives in the sample. To find the probability of accepting the lot (which means not more than 2 defectives in the sample), we must calculate P(X ≤ 2).
Since the calculations may require a graphing calculator or statistical software, we would need to use such tools to find:
P(X = 0) + P(X = 1) + P(X = 2)
Using the binomial distribution formula:
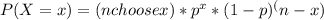
Each term represents the probability of getting exactly x defectives. The sum of these probabilities will give us the point on the OC curve for p = 0.06 and an acceptance number of 2.