To observe a 5.4% increase in the emitted frequency from a stationary sound source (343 m/s), an observer must approach with a speed that, when plugged into the Doppler formula, yields the desired frequency shift.
To determine the observed frequency (also known as Doppler frequency) when there is relative motion between a source and an observer, you can use the Doppler effect formula for frequency:
![\[ f' = (f \cdot (v + v_o))/((v + v_s)) \]](https://img.qammunity.org/2024/formulas/physics/high-school/j81wqekisnxj1hnpyivpxurtl87iusng4d.png)
Where:
- \( f' ) is the observed frequency,
- f is the emitted frequency by the source,
- v is the speed of sound,
-
 is the speed of the observer relative to the medium (positive if the observer is moving towards the source, negative if moving away),
is the speed of the observer relative to the medium (positive if the observer is moving towards the source, negative if moving away),
-
 is the speed of the source relative to the medium (positive if the source is moving away, negative if moving towards the observer).
is the speed of the source relative to the medium (positive if the source is moving away, negative if moving towards the observer).
In this case, the problem states that an observer needs to approach a stationary sound source in order to observe a 5.4% increase in the emitted frequency. The increase in frequency (\( \Delta f \)) can be related to the original frequency (\( f \)) by the formula:
![\[ \Delta f = (f' - f)/(f) * 100 \]](https://img.qammunity.org/2024/formulas/physics/high-school/hh1pjp3bmdimjv5rouu8j0pyneaijbfyc3.png)
Given that \( \Delta f = 5.4\% \), you can use this information to find \( f' \). Since the observer is approaching the source

 can be considered zero (stationary source).
can be considered zero (stationary source).
![\[ 5.4\% = (f' - f)/(f) * 100 \]](https://img.qammunity.org/2024/formulas/physics/high-school/x7rh2ua4qws1n189shsoclp9bufj4pu3xa.png)
Solving for

![\[ f' = f + 0.054 * f \]](https://img.qammunity.org/2024/formulas/physics/high-school/wif5bg3hnna6gtfg0e1cqua0rh26vqivt7.png)
Now you can substitute this into the Doppler formula:
![\[ f' = (f \cdot (v + v_o))/((v + v_s)) \]](https://img.qammunity.org/2024/formulas/physics/high-school/j81wqekisnxj1hnpyivpxurtl87iusng4d.png)
Substitute
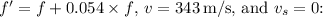
![\[ f + 0.054 * f = (f \cdot (343 + v_o))/((343 + 0)) \]](https://img.qammunity.org/2024/formulas/physics/high-school/c6bm4oh9ef2kfpkmbxvim1tgh4m9jmjmfm.png)
Now solve for
 , the speed of the observer. This will give you the speed the observer needs to approach the stationary sound source in order to observe a 5.4% increase in the emitted frequency.
, the speed of the observer. This will give you the speed the observer needs to approach the stationary sound source in order to observe a 5.4% increase in the emitted frequency.