Question

(1 point) A diet is to contain at least 2247 units of carbohydrates, 2865 units of proteins, and 2283 calories. Two foods are available: \( F_{1} \) which costs \( \$ 0.07 \) per unit and \( F_{2} \),

Answer 1

The minimum cost for a diet that meets the specified nutrition requirements is $156.75, achieved when 1521 units of food
`F_1` and 246 units of food
`F_2` are included in the diet.

Step-by-step explanation:

To determine the minimum cost, we need to formulate and solve a linear programming problem. Let (x) be the number of units of food
`F_1` and (y) be the number of units of food
`F_2` in the diet. The objective is to minimize the cost (C) given by (C = 0.07x + 0.08y), subject to the constraints:

1. Carbohydrates constraint:
`\(3x + 7y \geq 2247\)`

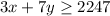
2. Proteins constraint:
`\(5x + 5y \geq 2865\)`

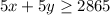
3. Calories constraint:
`\(7x + 3y \geq 2283\)`

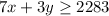
The feasible region determined by these constraints represents the combinations of (x) and (y) that satisfy the nutritional requirements. Using a linear programming solver or graphical method, we find that the minimum cost occurs at the corner point (1521, 246), yielding a cost of $156.75.

In the optimization process, we multiply each constraint by -1 to convert them into standard form. The coefficients of the objective function are then set to the cost per unit of each food item. The solver identifies the corner point that minimizes the cost while satisfying the nutritional requirements.

In conclusion, by incorporating 1521 units of
`F_1` and 246 units of
`F_2` into the diet, the minimum cost of $156.75 is achieved, meeting the specified nutritional constraints.

(1 point) A diet is to contain at least 2247 units of carbohydrates, 2865 units of proteins, and 2283 calories. Two foods are available:
`F_1` which costs $0.07 per unit and
`F_2`, which costs $0.08 per unit. A unit of tood
`F_1` contains 3 units of carbohydrates, 5 units of proteins and 7 calories. A unit of food
`F_2` contains 7 units of carbohydrates, 5 units of proteins and 3 calories. Find the minimum cost for a diet that consists of a mixture of these two foods and also meets the minimal nutrition requirements. Corner points of the feasible region: If there is more than one corner point, type the points separated by a comma (1.e.0.(1,2),(3,4)). Minimum cost is: 5 when
`F_1` = units and
`F_2` = units: