Jessica's account grows with compound interest at 222%. The expression
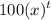 indicates
indicates
 as the factor by which $100 compounds annually. Here,
as the factor by which $100 compounds annually. Here,
 represents the multiplication factor for each year over
represents the multiplication factor for each year over
 years.
years.
Given:
Principal amount
 = $100
= $100
Annual interest rate
 = 222%
= 222%
The formula for compound interest is given by:
![\[ A = P * (1 + r)^t \]](https://img.qammunity.org/2024/formulas/business/high-school/q90581tnow7lfgd7ymp1rcjqwmc7yiuihh.png)
Where:
 is the final amount after
is the final amount after
 years
years
 is the principal amount (initial deposit)
is the principal amount (initial deposit)
 is the annual interest rate in decimal form
is the annual interest rate in decimal form
 is the time the money is invested for
is the time the money is invested for
First, convert the annual interest rate from percentage to decimal:
![\[ r = (222)/(100) = 2.22 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/g4emu2d38auk9w0emfbugf05df6z9jj3gr.png)
Now, the expression given is
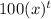 , suggesting that
, suggesting that
 is the factor by which the initial deposit
is the factor by which the initial deposit
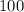 is multiplied each year for
is multiplied each year for
 years to find the final value of the account.
years to find the final value of the account.
Comparing the compound interest formula and the expression
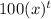 , we equate
, we equate
 with
with
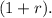
Therefore,
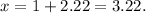
Thus, in the expression
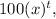 the value of
the value of
 is
is
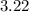 . This signifies that the initial deposit is multiplied by 3.22 each year for
. This signifies that the initial deposit is multiplied by 3.22 each year for
 years to calculate the account's final value.
years to calculate the account's final value.
complete the question
Jessica deposited $100 into a bank account that accrues compound interest at a rate of 222% annually. If she uses the expression $100(x)^t$ to represent the account value after \(t\) years, what is the value of \(x\) in the expression?