Final answer:
Function

 ssary alternating sign pattern, but the suitability for the unspecified part (IV) cannot be determined without additional information.
ssary alternating sign pattern, but the suitability for the unspecified part (IV) cannot be determined without additional information.
Step-by-step explanation:
The question seeks to find a holomorphic function at 0 that satisfies certain conditions based on the value of the function at 1/n for all n in the natural numbers
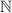 , we will address part (ii) and part (IV) of the question which are looking for the functions that satisfy:
, we will address part (ii) and part (IV) of the question which are looking for the functions that satisfy:
- (ii)
 n in
n in
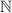
- (IV)
 o identify the correct function.
o identify the correct function.
To address part (ii), the function must alternate signs for successive values of n. A function that achieves this while being holomorphic at 0 is the function
 ot an option in the given set, so we need to find an equivalent function among the choices. The cosine function exhibits similar behavior, and
ot an option in the given set, so we need to find an equivalent function among the choices. The cosine function exhibits similar behavior, and
 ndidate since it alternates between -1 and 1 for integer values of z. Thus, the correct answer choice for part (ii) appears to be \( f(z) =
ndidate since it alternates between -1 and 1 for integer values of z. Thus, the correct answer choice for part (ii) appears to be \( f(z) =

However, without an explicit part (IV) condition provided, we cannot further analyze and confirm the suitability of the function for that part. Furthermore, we cannot guarantee this solution is correct for the entire set of conditions since the entirety of the question has not been provided. Therefore, while
 er (ii), its suitability for other parts is unclear without additional context.
er (ii), its suitability for other parts is unclear without additional context.