Question

Pulse rates. Suppose that a report by a leading medical organization claims that the healthy human heart beats an average of 72 times per minute. Advances in science have led some researchers to question if the healthy human heart beats an entirely different amount of time, on average, per minute. They obtain pulse rate data from a sample of 100 healthy adults and find the average number of heart beats per minute to be 75, with a standard deviation of 12. Before conducting a statistical test of significance, this outcome needs to be converted to a standard score, or a test statistic. What would that test statistic be?

(a) 3.0
(b) 2.5
(c) 0.25
(d) 0.03

Answer 1

The test statistic (z-score) is 2.5, suggesting a significant deviation of 2.5 standard deviations from the claimed average heart rate of 72 beats per minute in the sample of 100 healthy adults. So the option B is correct.

The test statistic, also known as the z-score, is calculated using the formula:

`\[ Z = \frac{{\text{{Sample Mean}} - \text{{Population Mean}}}}{{\text{{Standard Deviation of the Sample}} / \sqrt{\text{{Sample Size}}}}} \]`

In this case:

- Sample Mean
`(\(\bar{X}\))` = 75

- Population Mean
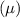`(\(\mu\))` = 72

- Standard Deviation
`(\(\sigma\))` = 12

- Sample Size
`(\(n\))` = 100

`\[ Z = \frac{{75 - 72}}{{12 / √(100)}} \]`

`\[ Z = \frac{{3}}{{1.2}} \]`

`\[ Z = 2.5 \]`

So, the correct answer is (b) 2.5.