Question

Where the dispersion isk = −t(cos(kxa)+cos(ky a)). Identify the energy where there is a van Hove singularity, and determine the leading energy dependence of the density of states near the van Hove singularity.

Answer 1

Van Hove singularities in the dispersion
`\( \varepsilon = -t(\cos(k_x a) + \cos(k_y a)) \) occur at \( \varepsilon = -2t \) or \( \varepsilon = 0 \). The leading DOS near these singularities is \( √(\varepsilon + 2t) \).`

The dispersion relation you provided is
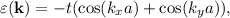`\( \varepsilon(\mathbf{k}) = -t(\cos(k_x a) + \cos(k_y a)) \),` where
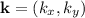`\( \mathbf{k} = (k_x, k_y) \)` is the wave vector,
`\( a \)` is the lattice constant, and
`\( t \)` is the hopping parameter.

A van Hove singularity occurs when the density of states (DOS) has a singularity due to the presence of a critical point in the band structure. In two dimensions, the van Hove singularities typically occur at points where the band structure has a saddle point. These points are located where the derivative of the dispersion relation with respect to \( k \) is zero.

For the given dispersion relation, the energy
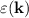`\( \varepsilon(\mathbf{k}) \)`is given by:

`\[ \varepsilon(\mathbf{k}) = -t(\cos(k_x a) + \cos(k_y a)) \]`

Now, let's find the critical points by taking the derivatives with respect to
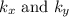`\( k_x \) and \( k_y \)` and setting them equal to zero:

`\[ (\partial \varepsilon)/(\partial k_x) = a t \sin(k_x a) = 0 \]`

`\[ (\partial \varepsilon)/(\partial k_y) = a t \sin(k_y a) = 0 \]`

These equations are satisfied when
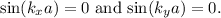`\( \sin(k_x a) = 0 \) and \( \sin(k_y a) = 0 \).`The solutions are
`\( k_x = n \pi/a \) and \( k_y = m \pi/a \), where \( n \) and \( m \) are integers.`

Now, let's find the energy at these critical points:

`\[ \varepsilon(k_x, k_y) = -t(\cos(n\pi) + \cos(m\pi)) \]`

Since
`\( \cos(n\pi) = (-1)^n \) and \( \cos(m\pi) = (-1)^m \)`, the energy at the critical points is:

`\[ \varepsilon(k_x, k_y) = (-1)^n t + (-1)^m t \]`

The van Hove singularities occur at points where either \( n \) or \( m \) is odd, resulting in
`\( \varepsilon = -2t \) or \( \varepsilon = 0 \).`

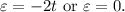
To determine the leading energy dependence of the density of states near the van Hove singularity, you can use the DOS formula:

`\[ \text{DOS}(\varepsilon) = \int (d^2k)/((2\pi)^2) \delta(\varepsilon - \varepsilon(\mathbf{k})) \]`

Near the van Hove singularity, you can approximate the dispersion relation as
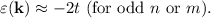`\( \varepsilon(\mathbf{k}) \approx -2t \) (for odd \( n \) or \( m \)).`In this case, the DOS will have a square root singularity:

`\[ \text{DOS}(\varepsilon) \propto √(\varepsilon + 2t) \]`

This is the leading energy dependence of the density of states near the van Hove singularity.