Question

Determine which of the following vector fields must be conservative. For each vector field that is conservative (i.e., has a potential function f), draw on the same set of axes, level curves that could represent the potential function f. Hint: what property do conservative vector fields have? Use this to show why some of these are not conservative by drawing certain paths. If f is a potential function for F then F = ∇f. Please use complete sentences to justify your answer.

Answer 1

None of the given vector fields is conservative because their curls are nonzero. Conservative vector fields have zero curl, ensuring independence of path in line integrals, a property crucial for potential functions.

To determine if a vector field is conservative, we need to check if its curl is zero. A vector field
`\(\mathbf{F}\)` is conservative if and only if
`\(\\abla * \mathbf{F} = \mathbf{0}\), where \(\\abla *\)`is the curl operator.

If
`\(\mathbf{F} = \langle M, N \rangle\), then the condition for \(\mathbf{F}\)`to be conservative is:

`\[(\partial N)/(\partial x) - (\partial M)/(\partial y) = 0\]`

Now, let's consider the vector fields given and determine which ones are conservative:

1.
`\(\mathbf{F} = \langle x, y \rangle\)`

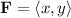
`\[\\abla * \mathbf{F} = (\partial)/(\partial x)(y) - (\partial)/(\partial y)(x) = 1 - (-1) = 2\]`

`Since \(\\abla * \mathbf{F}\) is not zero, \(\mathbf{F}\) is not conservative.`

2.
`\(\mathbf{F} = \langle -y, x \rangle\)`

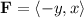
`\[\\abla * \mathbf{F} = (\partial)/(\partial x)(x) - (\partial)/(\partial y)(-y) = 1 - (-1) = 2\]`

Again,
`\(\\abla * \mathbf{F}\) is not zero, so \(\mathbf{F}\)`is not conservative.

3.
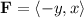`\(\mathbf{F} = \langle -y, x \rangle\)` (same as above)

This vector field is not conservative for the same reason as in example 2.

4.
`\(\mathbf{F} = \langle y, x \rangle\)`

`\[\\abla * \mathbf{F} = (\partial)/(\partial x)(x) - (\partial)/(\partial y)(y) = 1 - (-1) = 2\]`

Once again,
`\(\\abla * \mathbf{F}\) is not zero, so \(\mathbf{F}\)` is not conservative.

5.
`\(\mathbf{F} = \langle -y, -x \rangle\)`

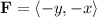
`\[\\abla * \mathbf{F} = (\partial)/(\partial x)(-x) - (\partial)/(\partial y)(-y) = -1 - 1 = -2\]`

This time,
`\(\\abla * \mathbf{F}\) is not zero, so \(\mathbf{F}\)` is not conservative.

Since none of the given vector fields have a curl of zero, none of them are conservative. Conservative vector fields have the property that their curl is zero, and this property is necessary for the existence of a potential function. Without a potential function, the line integral of the vector field becomes path-dependent, leading to different values for different paths between the same endpoints.