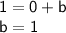Question

Find the equation of the line below

Answer 1

Choose any two points from the graph.

(0, 1) ; (3, -1)

• Using these points find the slope of the line.

`\sf \boxed{\bf Slope = (y_2-y_1)/(x_2-x_1)}`

`\sf = (-1-1)/(3-0)\\\\\\=(-2)/(3)`

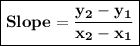
• Equation of the line in slope y-intercept form: y =mx + b

Here, m is the slope and b is the y-intercept. substitute m value in the above equation.

`y = (-2)/(3)x + b`

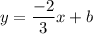
• The point (0,1) passes through the line. Substitute x =0 and y = 1 in the above equation and find the value of y-intercept.

`\sf 1 = 0 +b\\b = 1`

• Equation of the line:

`\boxed{\bf y =(-2)/(3)x+1}`