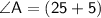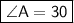Question

6.) Triangle ABC is isosceles.
What is the measure of angle A?

Answer 1

`{\boxed{\sf {\angle A = 30 \degree}}}`

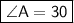
Explanation:

In isosceles triangle two congruent to each other and the two angles opposite to the equal sides are congruent to each other.

Equal sides = AB = AC

`\sf \therefore \angle B = \angle C`

`\sf \angle B = 3x`

`\sf \angle C = 3x`

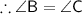
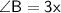
Also the sum of all the interior angles of the triangle is 180°.

`\sf \angle A + \angle B + \angle C = 180\degree`

`\sf x + 5 + 3x + 3x = 180`

`\sf x + 5 + 6x = 180`

`\sf 5 + 7x = 180`

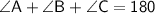
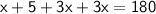
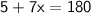
Subtract 5 from each side:

`\sf 7x = 175`

Divide 7 on both sides:

`\bf x = 25`

`\sf \angle A = (x + 5)`

`\sf \angle A = (25 + 5)`

`{\boxed{\sf {\angle A = 30 \degree}}}`