Question

Can some one please help me

Answer 1

The recursive formulas arranged in order from least to greatest are;

aₙ₊₁ = -5 + aₙ

↓

aₙ₊₁ = (-1/2)·aₙ

↓

`\underline{a_(n+1)=a_n + 1(2)/(3)}`

↓

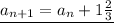
aₙ₊₁ = 2·aₙ

The steps used to find the correct order of the recursive formula can be presented as follows;

The 10th term of the recursive formula aₙ₊₁ = aₙ +
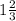`1(2)/(3)` is found as follows;

a₉₊₁ = a₉ +
`1(2)/(3)`

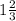
a₂ = a₁ +
`1(2)/(3)`

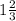
a₃ = a₂ +
`1(2)/(3)`

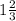
a₂ +
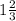`1(2)/(3)` = a₁ +
`1(2)/(3)` +
`1(2)/(3)`

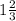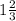
a₃ = a₁ + 2 ×
`1(2)/(3)`

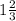
a₃ = a₁ + (3 - 1) ×
`1(2)/(3)`

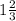
Similarly, a₉₊₁ = a₁ + (10 - 1) ×
`1(2)/(3)`

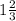
a₁₀ = -7
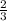`(2)/(3)` + (10 - 1) ×
`1(2)/(3)`

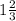
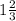`1(2)/(3)` = 5/3

-7
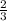`(2)/(3)` = -23/3

a₁₀ = (-23/3) + (10 - 1) × (5/3)

(-23/3) + (10 - 1) × (5/3) = 22/3

a₁₀ = 22/3

Similarly, we get; The 10th term of the formula aₙ₊₁ = -5 + aₙ, where a₁ = 32 is; a₁₀ = (10 - 1) × (-5) + 32

(10 - 1) × (-5) + 32 = -13

a₁₀ = -13

The formula aₙ₊₁ = 2·aₙ is a geometric progression, with the 10th term being a₁₀ = 2¹⁰ × a₁

The 10th term of the formula aₙ₊₁ = 2·aₙ, with a₁ = 0.125 is therefore;

a₁₀ = 2¹⁰ × 0.125

2 ¹⁰ × 0.125 = 128

a₁₀ = 128

The formula aₙ₊₁ = (-1/2)·aₙ is a geometric progression, with the 10th term being; a₁₀ = (-1/2)¹⁰ × a₁

a₁ = 2,048, therefore;

a₁₀ = (-1/2)¹⁰ × 2048

a₁₀ = 2