Answer:
112 m³
Explanation:
The given figure is a hemisphere with a slice removed, where the radius of the circular base of the hemisphere is 4 m, and the measure of the arc of the base of the removed slice is 60°.
Given that in a circle the measure of an arc is equal to the measure of its corresponding central angle, we can conclude that the central angle of the base of the removed slice is 60°.
Angles around a point sum to 360°. By dividing 60° by 360°, we find that the removed slice is equal to 1/6 of the entire hemisphere. Therefore, to find the volume of the figure, we need to find 5/6 of the volume of a hemisphere with radius 4 m.

Multiply the hemisphere formula by 5/6 and substitute r = 4:
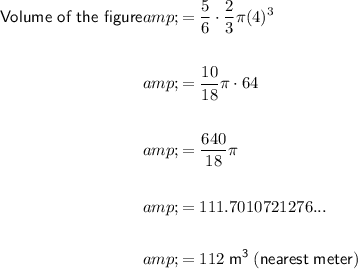
Therefore, the volume of the figure to the nearest meter is 112 m³.