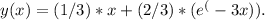Question

Consider the initial value problem y' = x-3y with y(0) = 1. (a) Solve the initial value problem to find a function y(x). (b) What is the equivalent integral equation for this problem?

(c) Show the steps or method for solving the equivalent integral equation.

Answer 1

The function y(x) for the initial value problem y' = x-3y with
`y(0) = 1 is y(x) = (1/3)*x + (2/3)*(e^(-3x))`
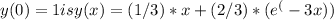
`y(x) = (1/3)*x + (2/3)*(e^(-3x)) + C`on y(0) = 1 into the integral equation, we can solve for the constant of integration C and find the specific function y(x).

Step-by-step explanation:

To solve the initial value problem y' = x-3y with y(0) = 1:

(a) We can find a function y(x) by solving the differential equation. Rearranging the equation, we get y' + 3y = x. To solve this, we can use an integrating factor. The integrating factor is e^(3x). Multiply the entire equation by this factor to get
`(e^(3x))*y' + 3*(e^(3x))*y = x*(e^(3x)).`
`(e^(3x))*y) = x*(e^(3x)).`h respect to x to obtain
`(e^(3x))*y = (1/3)*(x*(e^(3x))) +`gration. Divide both sides by (e^(3x)) to solve for y and we get
`y(x) = (1/3)*x + (C/(e^(3x))).`) = 1, we substitute x = 0 and y = 1 into the equation to find the value of C. Solving for C, we get C = 2/3. Therefore, the function y(x) is
`y(x) = (1/3)*x + (2/3)*(e^(-3x)).`

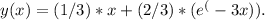
(b) The equivalent integral equation for this problem is
`y(x) = (1/3)*x + (2/3)*(e^(-3x)) + C.`

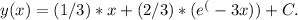
(c) To solve the equivalent integral equation, we can use the initial condition y(0) = 1. Substitute x = 0 and y = 1 into the equation to find the value of C. Solving for C, we get C = 2/3. Therefore, the function y(x) is
`y(x) = (1/3)*x + (2/3)*(e^(-3x)).`