Question

Supply and demand for commodity 1: 1. Supply of commodity 1: Qˢ₁= -6+2P₁​ 2. Demand for commodity 1: Qᴰ₁= 10-2P₁​+P₂ Supply and demand for commodity 2: 1. Supply of commodity 2: Qˢ₂= -2+3P₂ 2. Demand for commodity 2: Qᴰ₂= 3-2P₂+2P₁ You are required to answer the following questions: a) Find the equilibrium values for P₁ and P₂. b) Calculate the equilibrium quantities Q₁ and Q₂ at this equilibrium. c) Interpret the equilibrium values in the context of supply and demand for these two commodities.

Please provide a detailed explanation and show all steps to answer these questions. You may need to solve a system of equations to find the equilibrium values, and then use those values to calculate equilibrium quantities and interpret the results.

Answer 1

To find the equilibrium values for P1 and P2, use the supply and demand equations to set them equal to each other and solve the resulting system of equations. The equilibrium values are P1 = 3.25 and P2 = 2.3. To calculate the equilibrium quantities Q1 and Q2, substitute the equilibrium prices into the respective supply and demand equations. The equilibrium quantities are Q1 = 0.5 and Q2 = 4.9. The equilibrium values indicate a state of balance in the market, where quantity supplied equals quantity demanded for both commodities.

Step-by-step explanation:

To find the equilibrium values for P1 and P2, we need to solve the system of equations for the supply and demand of the two commodities. Start by setting the supply and demand equations equal to each other:

Qd1 = Qs1

`10-2P1+P2 = -6+2P1`

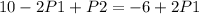
Now, solve this equation for P1:

1. Combine like terms: 10+6 = 2P1+2P1+P2
2. Combine like terms again: 16 = 4P1+P2
3. Use the second equation to substitute for P2: 16 = 4P1+(3-2P1+2P1)
4. Simplify: 16 = 4P1+3
5. Subtract 3 from both sides: 13 = 4P1
6. Divide by 4: P1 = 13/4 = 3.25

Now that we have P1, we can substitute it back into one of the equations to solve for P2:

Qs2 = -2+3P2

Qd2 = 3-2P2+2P1

Substitute P1 = 3.25 into the demand equation:

Qd2 = 3-2P2+2(3.25)

Simplify this equation:

1. Distribute the 2: Qd2 = 3-2P2+6.5
2. Combine like terms: Qd2 = 9.5-2P2

Now, set this equation equal to the supply equation and solve for P2:

Qd2 = Qs2

`9.5-2P2 = -2+3P2`

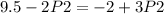
Combine like terms:

11.5 = 5P2

Divide both sides by 5:

P2 = 11.5/5 = 2.3

So the equilibrium values are P1 = 3.25 and P2 = 2.3.

To calculate the equilibrium quantities Q1 and Q2, substitute the equilibrium prices into the respective supply and demand equations:

Q1 = -6+2(3.25) = 0.5

Q2 = -2+3(2.3) = 4.9

In terms of supply and demand, the equilibrium values mean that at the equilibrium prices, quantity supplied equals quantity demanded for both commodities. This indicates that the market is in a state of balance, where there is neither excess supply nor excess demand. In this case, the equilibrium price and quantity for commodity 1 are P1 = 3.25 and Q1 = 0.5, and for commodity 2 are P2 = 2.3 and Q2 = 4.9.