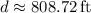Question

A lighthouse keeper is in the top of a lighthouse 95 feet above sea level. She notes that the angle of depression to a rock jutting just above the water is 6° . How far is the rock from the lighthouse?

Answer 1

808.72 ft

Explanation:

We can create a right triangle with the following points:

- the top of the lighthouse

- the base of the lighthouse

- the rock jutting out of the water

I will label the distance from the base of the lighthouse to the rock d.

See the attached image for a detailed model.

Then, solve for the two acute angles of the triangle.

The larger acute angle (∠1) is complementary to 6°, so we can solve by setting their sum to 90°:

6° + m∠1 = 90°

m∠1 = 84°

Because this is a right triangle, the smaller acute angle (∠2) is complementary to the larger acute angle, so we can solve once again by setting their sum to 90°:

m∠1 + m∠2 = 90°

84° + m∠2 = 90°

m∠2 = 6°

We can use either of these angles in combination with the trigonometric ratio tangent to solve for the distance d. I will use tangent of ∠1.

`\tan(84\textdegree) = \frac{d}{85 \, \textrm{ft}}`

`d = 85\tan(84\textdegree)`

`d \approx 808.72 \, \textrm{ft}`