Question

Create an equivalent expression for ( 1.4³ / 1.2⁴)⁻⁶

1.4³/1.2²
1.2²/1.4³
1.4¹⁶/1.2²⁴
1.2²⁴/1.4¹⁶

Answer 1

The correct answer is: [D]: "
`((1.2)^2^4)/((1.4)^1^8)` "

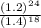Explanation:

Given: ( 1.4³ / 1.2⁴)⁻⁶ ;

Note the following properties of exponents:

1)
`a^-^b = (1)/(a^b)` ; {
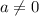`a\\eq 0` } ; and:

2) the 'power of a fraction' rule:

`((a)/(b))^c} =(a^c)/(b^c)` ;
`b\\eq 0` '

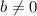As such:

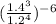`((1.4^3)/(1.2^4))^(-6)` ;

= 1 ÷ (
`(1.4^3)/(1.2^4))^(6)` ;

=
`(1)/(1)` ÷ (
`(1.4^3)/(1.2^4))^(6)` ;;

=
`(1)/(1)` ÷
`(1.4^(^3^*^6^))/(1.2^(^4^*^6^))` ;

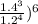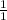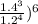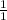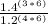Note the follow property of exponents:
"
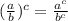`((a)/(b))^c = (a^c)/(b^c)` " ; {" b ≠ 0 "}.

⇒ =
`(1)/(1)` ÷
`(1.4^(^1^8^))/(1.2^(^2^4^))`

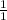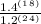
Note: Dividing by a fraction or a number; is the same as multiplying by the reciprocal (invert) of that number.

=
`(1)/(1)` *
`\frac{1.2^(^2^4^)}{{1.4^(^1^8^)}}` ;

= "
`\frac{1.2^(^2^4^)}{{1.4^(^1^8^)}}` " ;

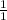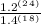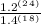which corresponds to:
Answer choice: [D]: "
`\frac{1.2^(^2^4^)}{{1.4^(^1^8^)}}` " .
____________
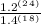Hope this is helpful! Best of luck!

____________