Question

Suppose that 11% of all steel shafts produced by a certain process are nonconforming but can be reworked (rather than having to be scrapped). Consider a random sample of 200 shafts, and let X denote the number among these that are nonconforming and can be reworked. (Round your answers to four decimal places.) (a) What is the (approximate) probability that X is at most 30

Answer 1

0.9726 = 97.26% approximate probability that X is at most 30

Explanation:

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

Can be approximated to a normal distribution, using the expected value and the standard deviation.

The expected value of the binomial distribution is:

`E(X) = np`

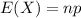
The standard deviation of the binomial distribution is:

`√(V(X)) = √(np(1-p))`

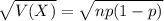
Normal probability distribution

Problems of normally distributed distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

When we are approximating a binomial distribution to a normal one, we have that
`\mu = E(X)`,
`\sigma = √(V(X))`.

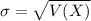11% of all steel shafts produced by a certain process are nonconforming but can be reworked (rather than having to be scrapped).

This means that
`p = 0.11`

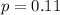
Random sample of 200 shafts

This means that
`n = 200`

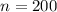
Mean and Standard deviation:

`\mu = E(x) = np = 200*0.11 = 22`

`\sigma = √(V(X)) = √(np(1-p)) = √(200*0.11*0.89) = 4.42`

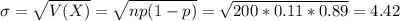
(a) What is the (approximate) probability that X is at most 30

Using continuity correction, this is
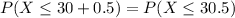`P(X \leq 30 + 0.5) = P(X \leq 30.5)`, which is the pvalue of Z when X = 30.5. So

`Z = (X - \mu)/(\sigma)`

`Z = (30.5 - 22)/(4.42)`

`Z = 1.92`

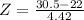
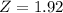
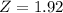`Z = 1.92` has a pvalue of 0.9726.

0.9726 = 97.26% approximate probability that X is at most 30