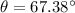Question

Pls community help meeee i want to sleep not stressing if im right or wrong i need help with the second one and for the first one tell me if I did it wrong

Answer 1

The ladder makes 75.43 degrees with the wall and the ground. To the nearest degree, the support wire makes 67 degrees with the pole and the ground.

Problem 2: A ladder leans against a wall

Let's denote the following:

-
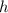`\( h \)`: height on the wall the ladder reaches (15 feet)

-
`\( b \)`: distance from the base of the ladder to the wall (3.9 feet)

-
`\( \theta \)`: the angle the ladder makes with the wall and the ground

Using trigonometry, specifically the tangent function, we can set up the following equation:

`\[ \tan(\theta) = (h)/(b) \]`

Substitute the given values:

`\[ \tan(\theta) = (15)/(3.9) \]`

Now, you can find
`\( \theta \)` by taking the arctangent (inverse tangent) of both sides:

`\[ \theta = \tan^(-1)\left((15)/(3.9)\right) \]`

`\theta= 75.43\textdegree`

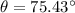
Problem 3: A support wire from a utility pole

Let's denote:

-
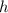`\( h \)`: height on the pole the wire reaches (7.2 meters)

-
`\( b \)`: distance from the base of the pole to the point on the ground (3 meters)

-
`\( \theta \)`: the angle the wire makes with the pole and the ground

Again, using trigonometry and the tangent function:

`\[ \tan(\theta) = (h)/(b) \]`

Substitute the given values:

`\[ \tan(\theta) = (7.2)/(3) \]`

Now, find
`\( \theta \)` by taking the arctangent of both sides:

`\[ \theta = \tan^(-1)\left((7.2)/(3)\right) \]`

`\theta= 67.38\textdegree`