Question

A manufacturer of potato chips would like to know whether its bag filling machine works correctly at the 417 gram setting. It is believed that the machine is underfilling the bags. A 13 bag sample had a mean of 415 grams with a standard deviation of 30. A level of significance of 0.1 will be used. Assume the population distribution is approximately normal. Determine the decision rule for rejecting the null hypothesis. Round your answer to three decimal places.

Answer 1

P value = 0.407

Hence, as P-value (0.407) is greater than 0.1

Fail to reject Null hypothesis

Explanation:

Given the data in the question;

sample size n = 13

sample mean x" = 415

standard deviation s = 30

level of significance = 0.1

so;

Null hypothesis H₀ : μ = 417

Alternative hypothesis Hₐ : μ < 417

degree of freedom DF = n - 1 = 13 -1 = 12

Rejection Region { level of significance = 0.1, df = 12 }

critical value of t is - 1.356 as { left tailed test }

Test Statistics;

t = (x" - μ) /
`(s)/(√(n) )`

we substitute

t = (415 - 417) /
`(30)/(√(13) )`

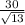
t = -2 /
`(30)/(√(13) )`

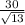
t = -0.24

so, from table;

P value = 0.407

Hence, as P-value (0.407) is greater than 0.1

Fail to reject Null hypothesis