Answer:
0.873 = 87.3% probability that an athlete tests negative and is not a user of an illegal drug
Explanation:
Conditional Probability
We use the conditional probability formula to solve this question. It is

In which
P(B|A) is the probability of event B happening, given that A happened.
 is the probability of both A and B happening.
is the probability of both A and B happening.
P(A) is the probability of A happening.
In this question:
Event A: Not a user of an illegal drug.
Event B: Tests negative.
The test for this drug is 90% accurate for non-drug users (this means there is a 90% chance the test comes back negative if the person is a non-user)
This means that
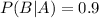
It is estimated that 3% of the athletes competing in a large tournament are users of an illegal drug to enhance performance.
So 100 - 3 = 97% probability that the athlete does not use the drug, so
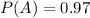
What is the probability that an athlete tests negative and is not a user of an illegal drug

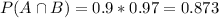
0.873 = 87.3% probability that an athlete tests negative and is not a user of an illegal drug