Question

A body moves on a coordinate line such that it has a position sequals​f(t)equalstsquaredminus4tplus3on the interval 0less than or equalstless than or equals7​,with s in meters and t in seconds.a. Find the​ body's displacement and average velocity for the given time interval.b. Find the​ body's speed and acceleration at the endpoints of the interval.c.​ When, if​ ever, during the interval does the body change​ direction?

Answer 1

A) Δf = - 49 m, B) v (7) = -56 m / s, a = - 8 m / s², C) t = 0.866 s

Step-by-step explanation:

A) In this exercise ask to find the displacement and the average velocity, give the function of the movement

f (t) = - 4t² +3

and the range of motion 0≤ t ≤ 7

the displacement is

for t = 0

f (0) = 3

for t = 7 s

f (7) = - 4 7² +3

f (7) = -46 m

the total displacement is

Δf = f (7) - f (0)

Δf = -46 - 3

Δf = - 49 m

the average speed is defined as the displacement between the time interval

v = Df / Dt

v = -49 / 7

v = - 7 m / s

B) the speed and acceleration of the end points of the motion

the speed of defined by

v =
`(dx)/(dt)`

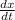
in this case

v =
`(df)/(dt)`

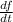
v = -8t

let's calculate

v (7) = -8 7

v (7) = -56 m / s

acceleration is defined by

a =
`(dv)/(dt)`

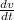
a = - 8 m / s²

acceleration is constant throughout the movement

C) the point where the direction changes.

This point is a point where the position goes from positive to negative, the point f = 0

0 = -4t² +3

t = √¾

t = 0.866 s