Question

Select all of the inequalities that could be represented by the graph. 2 y + 5 > = (x+3) 3 Y> 2 x - 7 -2x - 3y < 21 -2x - 3y > 21 y + 11 > 2 -Y> x+7 (x − 6) -​

Answer 1

The inequalities that could be represented by the graph include:

B. y > -2/3(x) - 7

C. -2x - 3y < 21

E. y + 11 > -2/3(x - 6).

In Mathematics and Geometry, the point-slope form of a straight line can be calculated by using the following mathematical equation (formula):

`y - y_1 = m(x - x_1)`

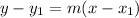
Where:

• x and y represent the data points.
• m represent the slope.

First of all, we would determine the slope for the line by using these points (0, -7) and (-6, -3);

`Slope(m)=(y_2-y_1)/(x_2-x_1)`

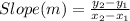
Slope (m) = (-3 + 7)/(-6 - 0)

Slope (m) = -4/6

Slope (m) = -2/3

At data point (0, -7) and a slope of -2/3, an equation for this line can be calculated by using the point-slope form as follows:

y + 7 = -2/3(x - 0)

y = -2/3(x) - 7

y > -2/3(x) - 7 (since the line is dashed and shaded above).

By rewriting the inequality in standard form, we have;

3y > -2x - 21

2x + 3y > -21

-2x - 3y < 21

Since the dashed also passed through the point (6, -11), we have the following inequality in point-slope form;

y + 11 > -2/3(x - 6)

Complete Question;

Select all of the inequalities that could be represented by the graph.

y+5> 2/3(x+3)

y>- 2/3(x)-7

-2x-3y<21

-2x-3y>21

y+11>-2/3(x-6)

-y> 2/3(x)+7