Question

You are removing branches from your roof after a big storm. You throw a branch horizontally from your roof, which is a height 3.00 m above the ground. The branch lands a horizontal distance 8.00 m away from where you threw it (assuming you are the 0 position in x, and the branch traveled in the x direction). You can assume there is no air resistance. You can assume that the upwards direction is positive. What is the initial velocity in x of the branch (how fast did you throw the branch)

Answer 1

The initial velocity in the x-direction with which the branch was thrown is approximately 10.224 m/s

Step-by-step explanation:

The given parameters of the motion of the branch are;

The height from which the branch is thrown = 3.00 m

The horizontal distance the branch lands from where it was thrown, x = 8.00 m

The direction in which the branch is thrown = Horizontally

Therefore, the initial vertical velocity of the branch,
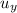`u_y` = 0 m/s

The time it takes an object in free fall (zero initial downward vertical velocity) to reach the ground is given as follows;

s =
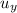`u_y`·t + 1/2·g·t²

Where;

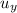`u_y` = 0 m/s

s = The initial height of the object = 3.00 m

g = The acceleration due to gravity = 9.8 m/s²

∴ s = 0·t + 1/2·g·t² = 0 × t + 1/2·g·t² = 1/2·g·t²

t = √(2·s/g) = √(2 × 3/9.8) = (√30)/7 ≈ 0.78246

The horizontal distance covered before the branch touches the ground, x = 8.00 m

Therefore, the initial velocity in the horizontal, x-direction with which the branch was thrown, 'uₓ', is given as follows;

uₓ = x/t = 8.00 m/((√30)/7 s)

Using a graphing calculator, we get;

uₓ = 8.00 m/((√30)/7 s) = (28/15)·√30 m/s ≈ 10.224 m/s

The initial velocity in the horizontal, x-direction with which the branch was thrown, uₓ ≈ 10.224 m/s.