Question

Find the greatest common factor of 27p^2q^3, 45p^3q^4, 9p^4q^3.

Answer 1

The greatest common factor of the given expressions is
`9p^2q^3.`

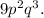
Step-by-step explanation:

To find the greatest common factor of
`27p^2q^3`^4, and
`9p^4q^3`ed to determine the highest power of each variable that appears in all three expressions. Let's break down each expression:

`27p^2q^3 = 3^3 * p^2 * q^3`

`45p^3q^4 = 3^2 * 5 * p^3 * q^4`

`9p^4q^3 = 3^2 * p^4 * q^3`

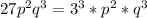
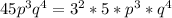
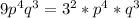
We can see that the highest power of 3 that appears in all three expressions is
`3^2`imilarly, the highest power of p is
`p^2`d the highest power of q is
`q^3`herefore, the greatest common factor is
`3^2 * p^2 * q^3 = 9p^2q^3.`