Question

A brine solution of salt flows at a constant rate of 4 L/min into a large tank that initially held 100 L of pure water. The solution inside the tank is kept well stirred and flows out of the tank at a rate of 3 L/min. If the concentration of salt in the brine entering the tank is 0.2 kg/L, determine the mass of salt in the tank after t min. When will the concentration of salt in the tank reach 0.1 kg/L?

Answer 1

a.
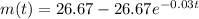`m(t) = 26.67 - 26.67e^(-0.03t)` b. 7.44 s

Explanation:

a. If the concentration of salt in the brine entering the tank is 0.2 kg/L, determine the mass of salt in the tank after t min.

Let m(t) be the mass of salt in the tank at any time, t.

Now, since a brine solution flows in at a rate of 4 L/min and has a concentration of 0.2 kg/L, the mass flowing in per minute is m' = 4 L/min × 0.2 kg/L = 0.8 kg/min

Now, the concentration in the tank of volume 100 L at any time, t is m(t)/100 L. Since water flows out at a rate of 3 L/min, the mass flowing out per minute is

m(t)/100 × 3 L/min = 3m(t)/100 kg/min

Now the net rate of change of mass of salt in the tank per minute dm/dt = mass flowing in -mass flowing out

dm/dt = 0.8 kg/min - 3m(t)/100 kg/min

So, dm/dt = 0.8 - 0.03m(t)

The initial mass of salt entering m(0) = 0 kg

dm/dt = 0.8 - 0.03m(t)

separating the variables, we have

dm/[0.8 - 0.03m(t)] = dt

Integrating, we have

∫dm/[0.8 - 0.03m(t)] = ∫dt

-0.03/-0.03 × ∫dm/[0.8 - 0.03m(t)] = ∫dt

1/(-0.03)∫-0.03dm/[0.8 - 0.03m(t)] = ∫dt

-1/0.03㏑[0.8 - 0.03m(t)] = t + C

㏑[0.8 - 0.03m(t)] = -0.03t - 0.03C

㏑[0.8 - 0.03m(t)] = -0.03t + C' (C'= -0.03C)

taking exponents of both sides, we have

`0.8 - 0.03m(t) = e^(-0.03t + C') \\0.8 - 0.03m(t) = e^(-0.03t)e^(C')\\0.8 - 0.03m(t) = Ae^(-0.03t) A = e^(C')\\0.03m(t) = 0.8 - Ae^(-0.03t)\\m(t) = 26.67 - (A)/(0.03) e^(-0.03t)\\when t = 0 \\m(0) = 0\\m(0) = 26.67 - (A)/(0.03) e^(-0.03(0))\\\\0 = 26.67 - (A)/(0.03) e^(0)\\26.67 = (A)/(0.03) \\(A)/(0.03) = 26.67\\(A)/(0.03) = 6.67\\A = 26.67 X 0.03\\A = 0.8\\m(t) = 26.67 - (A)/(0.03) e^(-0.03t)\\\\m(t) = 26.67 - (0.8)/(0.03) e^(-0.03t)\\`

So, the mass of the salt after t min is

`m(t) = 26.67 - 26.67e^(-0.03t)`

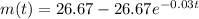
b. When will the concentration of salt in the tank reach 0.1 kg/L?

When the concentration of the salt reaches 0.1 kg/L, m(t) = 0.1 kg/L

Solving the equation for t,

`m(t) = 26.67 - 6.67e^(-0.03t)\\0.1 = 26.67 - 26.67e^(-0.03t)\\26.67e^(-0.03t) = 26.67 - 0.1\\26.67e^(-0.03t) = 26.57\\e^(-0.03t) = 26.56/26.67\\e^(-0.03t) = 0.9963\\`

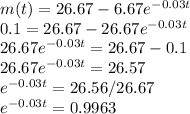
taking natural logarithm of both sides, we have

-0.03t = ㏑0.9963

-0.03t = -0.0038

t = -0.0038/-0.03

t = 0.124 min

t = 0.124 × 60 s

t = 7.44 s