If the same combined abatement level of 14 units is to be met in a cost-effective manner, the standards for each should be: (A_O = 4.8; A_N = 7.2). (Option B)
How is that so?
Combined Abatement Level: We need to achieve a total abatement of 14 units, regardless of the source. So,
 .
.
Cost-Effectiveness: In a cost-effective scenario, the marginal abatement costs (MAC) should be equal for both sources. This means:
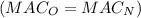
Substituting the given functions:

Solving the System: We have two equations with two unknowns: (A_O) and
 . We can solve this system simultaneously using substitution or elimination.
. We can solve this system simultaneously using substitution or elimination.
Here's the solution using substitution:
- From the first equation (combined abatement):
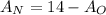
- Substitute this expression into the second equation (equal MACs):
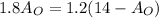
- Solve for
 :
:

- Find

- Calculate

Checking the Options: Only option B satisfies both conditions:
 and
and
 (rounded to 7.2 for easier comparison)
(rounded to 7.2 for easier comparison)
Substituting these values into the MAC equations:
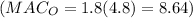
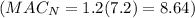
Therefore, setting the standards to
 and
and
 achieves the desired combined abatement level (14 units) with equal marginal abatement costs for both sources, making it the most cost-effective solution.
achieves the desired combined abatement level (14 units) with equal marginal abatement costs for both sources, making it the most cost-effective solution.