Question

Find the equation of the line in the form ax + by =c, passing through ( - 12,27) and parallel to 9x + 4y = 17

Answer 1

9x + 4y = 0

Explanation:

the equation of a line in slope- intercept form is

y = mx + c ( m is the slope and c the y- intercept )

given

9x + 4y = 17 ( subtract 9x from both sides )

4y = - 9x + 17 ( divide through by 4 )

y = -
`(9)/(4)` +
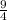`(17)/(4)` ← i slope- intercept form

with slope m = -
`(9)/(4)`

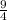
• Parallel lines have equal slopes , then

y = -
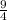`(9)/(4)` x + c ← is the partial equation

to find c, substitute (- 12, 27 ) for x and y in the partial equation

27 = -
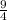`(9)/(4)` (- 12) + c = 27 + c ( subtract 27 from both sides )

0 = c

y = -
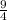`(9)/(4)` x + 0 ← equation in slope- intercept form

multiply through by 4

4y = - 9x + 0 ( add 9x to both sides )

9x + 4y = 0 ← equation of parallel line in form ax + by = c

Answer 2

The equation of the line parallel to 9x + 4y = 17 and passing through (-12, 27) in the form ax + by = c is -9x + 4y = -216.

How to find the equation of the line?

Step 1: The given line is 9x + 4y = 17. To find the slope, rearrange the equation into the slope-intercept form (y = mx + b) where m is the slope:

4y = -9x + 17

y = -9/4x + 17/4

So, the slope (m) is -9/4.

Step 2: Since the desired line is parallel, it has the same slope: m = -9/4.

Step 3: Use the point-slope form
`(\(y - y_1 = m(x - x_1)\))` with the point (-12, 27) and the slope -9/4:

`\[ y - 27 = -(9)/(4)(x + 12) \]`

Step 4: Convert to the standard form ax + by = c:

-9x + 4y = -216